Knowledge Hunt

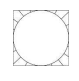
 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 26 Find the area of the unshaded region shown in the given figure.  Solution: modified view of shared image The total horizontal or vertical extent of the region is 8 cm and extent includes the side length of the square (𝑎)  and the diameters of the semicircles on either side of the square. Given that each semicircle has a radius 𝑟, the side length of the square is 𝑎=8−2𝑟. Given the figure, the radius 𝑟 of each semicircle is 2cm Side Length of the Square 𝑎 = 8-2x2  = 4 cm Area of the Square:  𝑎^2 = 4^2 = 16 Area of One Semicircle = \[ \frac{1}{2} {\pi} r^2 = 2{\pi} \; cm^2\; where \; r= 2 \] Combined Area of Four Semicircles: \[4\times 2\pi\; cm^2\] Area of the unshaded region=Area of the square + 4 x Area of  one semicircle = \[16 \; cm^2 + 4 \times2 {\pi} \; cm^2 \] \[(\;16 + 8{\pi}\;) \; cm^2\] 

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 25 Find the value of x if \begin{flalign} & 2\;cosec^2(30) + xsin^2(60)-\frac{3}{4}tan^2(30)= 10 &\ \end{flalign} Explanation:    Now simplify the equation below  \begin{flalign} & 2\;cosec^2(30) + xsin^2(60)-\frac{3}{4}tan^2(30)= 10 &\\ \end{flalign} \[ \Rightarrow 2\times(2)^2+ x\left(\frac{\sqrt{3}}{2}\right)^2 -\frac{3}{4}\left(\frac{1}{\sqrt{3}}\right)^2 = 10 \] we know that value of cosec(30) , sin(60)and tan(30) putting their values \[ \Rightarrow 2\times 4 + x\frac{3}{4} - (\frac{3}{4}\times\frac{1}{3} )= 10 \] \[ OR \; 8 + \frac{3x}{4} - \frac{1}{4} = 10 \] \[ OR \; \frac{3x}{4} = \frac{1}{4} +(10-8) \] \[ OR \; \frac{3x}{4} = \frac{1}{4} +2 \Rightarrow \frac{9}{4} \] \[ OR \; \frac{3x}{4} = \frac{9}{4} \] \[\Rightarrow {3x} = {9} \] \[\therefore{x} = {3} \]

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041)  Question 24 \[if\; \tan(A + B) =\;\sqrt{3} \; and \;\tan(A - B) =\;\frac{1}{\sqrt{3}}\] , 0° < A + B < 90°; A > B, find A and B. Explanation:   Given that : \[ \tan(A + B) =\;\sqrt{3} \; and \;\tan(A - B) =\;\frac{1}{\sqrt{3}}\] First, recognize the angles for which \[\tan(\theta) = \sqrt{3} \; and \; tan(\theta) = \frac{1}{\sqrt{3}}\] we know that \[\tan(60^{\circ}) = \sqrt{3} \; and \; tan(30^{\circ}) = \frac{1}{\sqrt{3}}\] \[\ so,\; A+B \;= 60^{\circ} \; and \; A-B \;= 30^{\circ}\] now add the two equation , we get  \[\ A+B \;= 60^{\circ},---(1)\]  \[\ A-B \;= 30^{\circ},---(2)\] \[\ so,\;(A+B)+(A-B)\;= 60^{\circ} + 30^{\circ}\] \[\ or,\;2A\;= 90^{\circ}\] \[\ or,\;A\;= 45^{\circ}\] Now Put A value in equation (1) and we get  \[\ from (1),\;B\;= 60^{\circ} - 45^{\circ}\] \[\therefore \; B\;= 15^{\circ}\] \[Now \; A\;= 45^{\circ}\; and \; B\;= 15^{\circ}\...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) SECTION B - Question 23 From an external point P, two tangents, PA and PB are drawn to a circle with centre O. At a point E on the circle, a tangent is drawn to intersect PA and PB at C and D, respectively. If PA = 10 cm, find the perimeter of ∆PCD . . Explanation: Given an external point  𝑃 from which two tangents 𝑃𝐴   and 𝑃𝐵 are drawn to a circle with center 𝑂, we know the following: 𝑃𝐴 = 10 cm PB=10 cm (since the tangents drawn from an external point to a circle are equal) \[\ Perimeter \;of\; ΔPCD = PC + CD + PD \] \[\Rightarrow PC + CE + ED + PD \] \[\Rightarrow PC + CA + DB + PD \] \[\Rightarrow PA + PB \] \[\Rightarrow PA + PB\] \[OR PA + PA\] \[OR 2PA\] \[= 2(10)\] \[ Perimeter \;of\; ΔPCD \; is \; 20 \; cm,\; where \;CE = CA, \;DE = DB,\; PA = PB \] Note: Tangents from the internal point to a circle are equal

MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) SECTION B -  Question 22 Prove that √2 is an irrational number.  Proof: Let us assume  √2  is a rational number. Then, there exist positive integers a and b such that \[\sqrt{2} =\frac{p}{q} where,\; p\; and\; q,\; are \;co-prime\; i.e. \;their\; HCF \;is \;1\] \[ OR \; (\sqrt{2})^2 =\frac{p^2}{q^2} \] \[OR\; 2 =\frac{p^2}{q^2} \] \[OR\; 2 \times q^2 = p^2 \] This implies that 𝑝^2 is even because it is equal to 2𝑞^2. From this, we can conclude that 𝑝 must also be even because the square of an odd number is odd, and the square of an even number is even. Let 𝑝 = 2k, where k is arbitrary integer , Substituting 𝑝 = 2k into the equation 2q^2 = p^2 we get  Substituting 𝑝 = 2k into the equation 2q^2 = p^2 \[\Rightarrow 2q^2 = (2k)^2 \] \[\Rightarrow 2q^2 = 4(k)^2 \] \[\Rightarrow q^2 = 2(k)^2 \] This implies that 𝑞^2  is even. Therefore, 𝑞 must also be even. Now...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) SECTION B -  Question 21 ABCD is a parallelogram. Point P divides AB in the ratio 2:3 and point Q divides DC in the ratio 4:1. Prove that OC is half of OA. Explanation : ABCD is a parallelogram. we know that AB = DC let AB = l then AB = DC = l given that Point P divides AB in the ratio 2:3 \[\Rightarrow AP = \frac{2}{5}\times l;and\;BP \;=\frac{3}{5}\times l \] \[Since\; we \;considered\; AP + BP = AB = l \] \[\Rightarrow DQ = \frac{4}{5}\times l;and\;CQ \;=\frac{1}{5}\times l \] given that point Q divides DC in the ratio 4:1 \[Since \; DQ + CQ = DC= l \] As we know that [AA similarity] \[\therefore ∆ APO \thicksim ∆ CQO \] \[\therefore \frac{AP}{CQ} = \frac{PO}{QO} = \frac{AO}{CO} \] \[\therefore \frac{AO}{CO} = \frac{\frac{2}{5}\times l}{\frac{1}{5}\times l} = \frac{2}{1} \] After simplifying the above equation, we get \[\Rightarrow OC = \frac{1}{2} \times AO \]

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 20 \begin{flalign} & Statement A (Assertion )\; 5,\; \frac{-5}{2},\; 0,\; \frac{5}{2} …. \;is\; in \;Arithmetic\; Progression.&\\ \end{flalign} Statement R (Reason) : The terms of an Arithmetic Progression cannot have both positive and negative rational numbers. (a) Both assertion (A) and reason (R) are true and reason (R) is the correct explanation of assertion (A) (b) Both assertion (A) and reason (R) are true and reason (R) is not the correct explanation of assertion (A) (c) Assertion (A) is true but reason (R) is false. (d) Assertion (A) is false but reason (R) is true. Explanation:   let's understand the AP where AP is a sequence of numbers in order, in which the difference between any two consecutive numbers is a constant value. The general form of an arithmetic progression is a , a+d,a+2d,a+3d,… where  a is the first term . d is the common differe...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 19 DIRECTION: In the question number 19 and 20, a statement of assertion (A) is followed by a statement of Reason (R). Choose the correct option Statement A (Assertion): Total Surface area of the top is the sum of the curved surface area of the hemisphere and the curved surface area of the cone. Statement R( Reason) : Top is obtained by joining the plane surfaces of the hemisphere and cone together (a) Both assertion (A) and reason (R) are true and reason (R) is the correct explanation of assertion (A)  (b) Both assertion (A) and reason (R) are true and reason (R) is not the correct explanation of assertion (A) (c) Assertion (A) is true but reason (R) is false. (d) Assertion (A) is false but reason (R) is true.  Explanation :  (b) Both assertion (A) and reason (R) are true and reason (R) is not the correct explanation of assertion (A) Statement A (Assertion) is true becaus...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 18 The upper limit of the modal class of the given distribution is: (a) 165  (b) 160  (c) 155  (d) 150 Explanation :  The class interval  Below 165 has the highest frequency of 51 girls. To find the upper limit of this class interval, we consider that the class interval "Below 165" starts from 160 and ends at the upper limit of the class interval before it. So, the upper limit of the modal class Below 165 is Upper limit = 165  Upper limit=165. Guess the Option and comment below   👇

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 17 2 cards of hearts and 4 cards of spades are missing from a pack of 52 cards. A card is drawn at random from the remaining pack. What is the probability of getting a black card? \begin{flalign} (a)\;\;& \frac{22}{52}\\ (b)\;\;& \frac{22}{46}&\\ (c)\;\;& \frac{24}{52}&\\ (d)\;\;& \frac{24}{46}&\\ \end{flalign} Explanation :  let's first determine the number of black cards in the original pack, and then subtract the number of hearts and spades removed. In a standard deck of 52 cards, there are 26 black cards (13 spades and 13 clubs), 13 hearts, and 13 diamonds. \[total\; number\; of\; remaining\; cards\; is\; = \; 11 + 9 + 13 + 13 \] After removing 2 hearts and 4 spades, we are left with 11 hearts (13 - 2) 9 spades (13 - 4) 13 clubs (unchanged) 13 diamonds (unchanged) \[total\; number\; of\; remaining\; cards\; is\; = \; 11 + 9 + 13 + 13 \] there are 22 ...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 16 There is a square board of side ‘2a’ units circumscribing a red circle. Jayadev is asked to  keep a dot on the above said board. The probability that he keeps the dot on the shaded  region is. \begin{flalign} (a)\;\;& \frac{\pi}{4}\\ (b)\;\;& \frac{4-\pi}{4}&\\ (c)\;\;& \frac{\pi - 4}{4}&\\ (d)\;\;& \frac{4}{\pi}&\\ \end{flalign} Explanation :  Given that , The square board has side length 2a  units and the board circumscribes a red circle. The shaded region represents the area within the square board but outside the red circle. so we have to find the ratio of the area of the shaded region to the total area of the square board. we know that area of the square is side^2  => 4a^2 square units. similarly  area of the red circle   => π(radius)^2 = π(a)^2 \[\therefore Area \; of\; shaded\; r...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 15 It is proposed to build a new circular park equal in area to the sum of areas of two circular parks of diameters 16 m and 12 m in a locality. The radius of the new park is (a) 10m  (b) 15m  (c) 20m  (d) 24m Explanation :  we first need to calculate the areas of the two circular parks with diameters of 16 m and 12 m, respectively.Then, we can find the sum of these areas. Let's calculate \[ Area \; of\; the\; circular\; park\; with\; diameter \;16 m\; =\; \pi\times \frac{16^2}{2^2} = \pi\times 8^2 m^2 \] \[ Area \; of\; the\; circular\; park\; with\; diameter \;12 m\; =\; \pi\times \frac{12^2}{2^2} = \pi\times 6^2 m^2 \] \[ Now,\; we \;find\; the \; Total \;Area\; =\; \pi\times 64 + \pi\times 36 \; = 100 \pi m^2 \] To find the radius of the new park with an area equal to 100𝜋m^2 , we use the formula for the area of a circle  => Total area =  𝜋 r^2...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 14 If the perimeter and the area of a circle are numerically equal, then the radius of the circle is (a) 2 units  (b) π units  (c) 4 units  (d) 7 units Explanation :  This question is really easy to answer. The perimeter of a circle (circumference) is given by 2𝜋𝑟 where 𝑟 is the radius. The area of a circle is given by 𝜋𝑟^2, where 𝑟 is the radius Given that the perimeter and the area are numerically equal, we can set up the equation \[ given \; that\; {2πr \; = 2π(r)^2} \] To solve for 𝑟, we can divide both sides of the equation by \[ \Rightarrow  {r} \; = 2 \; units \] Guess the Option and comment below   👇

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041)  Question 13 If a pole 6 m high casts a shadow 2 √3m long on the ground, then the Sun’s elevation is (a) 60°  (b) 45°  (c) 30°  (d) 90° Explanation :  let's assume  h as the height of the pole (6 meters) s as the length of the shadow (2√3 meters) θ as the Sun's elevation angle The triangles formed by the pole, its shadow, and the ground are similar. Therefore, the ratio of corresponding sides is equal. In this case, the ratio of the height of the pole to the length of its shadow is  the same as the ratio of the height of the observer to the distance from the observer to the tip of the shadow. \[\therefore \frac{h}{s} \; = \tan\theta\] \[\Rightarrow \frac{6}{2\sqrt{3}} \; = \tan\theta\] \[\therefore \sqrt{3} \; = \tan\theta\] \[\theta\; =\; \sqrt{3}\] The above question is illustrated in the picture below as well  : Where X =  2√3 and θ =...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041)  Question 12 (sec A + tan A) (1 – sin A) equals : (a) sec A  (b) sin A  (c) cosec A  (d) cos A Explanation :  let's first express  sec A and tan A in terms of sinA and cos A We know that  \[sec A \; =\; \frac{1}{cos A }\]  \[tan A \; =\; \frac{sin A}{cos A }\]  \[(secA+tanA)(1−sinA) \; = (\frac{1}{cosA} + \frac{sinA}{cosA}) \;(1−sinA) \] \[\Rightarrow (\frac{1+sinA}{cosA})(1−sinA) \] Now, let's expand this expression \[\Rightarrow \frac{(1+sinA)(1-sinA)}{cosA} \] \[\Rightarrow \frac{(1-sin^2A)}{cosA} \] \[we\; know \;that \; { 1-cos^2A} \;=\; sin^2A, 💡  \] \[\therefore \frac{(cos^2A)}{cosA} \; =\; cosA \] Guess the Option and comment below   👇

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041)  Question 11 \begin{flalign} & Given \; that \; \sin\theta \; = \; \frac{a}{b},then \; \cos\theta\ is\;? &\\ \end{flalign} \begin{flalign} (a)\;\;& \frac{b}{\sqrt{b^2 - a^2}}\\ (b)\;\;& \frac{b}{a} \\ (c)\;\;& \frac{\sqrt{b^2 - a^2}}{b}\\ (d)\;\;& \frac{a}{\sqrt{b^2 - a^2}}&\\ \end{flalign} Explanation :  \begin{flalign} & Given \; that \; \sin\theta \; = \; \frac{a}{b},to \; find \;the \; \cos\theta &\\ \end{flalign} The Pythagorean identity states that in a right triangle, the square of the length of the hypotenuse (c) is equal to the sum of the squares of the lengths of the other two sides (𝑎 and 𝑏).  Mathematically, it is represented as  \[ \sin^2\Theta + \cos^2\Theta = 1, -----(a) \]  \begin{flalign} & Since \; we \; know\; that\; \sin\theta \; = \; \frac{a}{b} &\\ \end{flalign} we can substitute this value into the Pyth...

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041)  Question 10   A quadrilateral PQRS is drawn to circumscribe a circle the tangent  If PQ = 12 cm, QR = 15 cm and RS = 14 cm, then find the length of SP is (a) 15 cm  (b) 14 cm (c) 12 cm  (d) 11 cm Explanation :  A circle is inscribed inside the quadrilateral, such that each side of the quadrilateral is tangent to the circle.  Now, in a cyclic quadrilateral, the opposite angles are supplementary Given that PQRS is a cyclic quadrilateral,  \[ \therefore PQ + RS = QR + SP,-----(a) \] Given that  PQ=12 cm QR=15 cm RS=14 cm  and putting eq(a) \[ \therefore 12+14=15+SP \] \[ \therefore SP=26−15 \] \[ \therefore SP=11 \] Guess the Option and comment below   👇

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041)  Question 9  If O is centre of a circle and Chord PQ makes an angle 50° with the tangent PR at the point of contact  P, then the angle subtended by the chord at the centre is (a) 130°  (b) 100°                                                               (c) 50°    (d) 30° Explanation :  OP ⊥ PR   [.. it indicates that the line segment 𝑂𝑃 is perpendicular to the line 𝑃𝑅 ] ∠OPQ = 90° – 50° = 40° OP = OQ   ...[Radii] ∴ ∠OPQ = ∠OQP = 40° In ΔOPQ,  ∠POQ + ∠OPQ + ∠OQP = 180° [.. applied the angle sum property ]  ∠POQ + 40° + 40° = 180°  ∠POQ = 180° – 80° = 100° Guess the Option and comment below   👇

 MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041)  Question 8  In 𝛥 ABC, DE ‖ AB. If AB = a, DE = x, BE = b and EC = c Then x expressed in terms of a, b and c is: \begin{flalign} (a)\;\;& \frac{ac}{b}\\ (b)\;\;& \frac{ac}{b+c} \\ (c)\;\;& \frac{ab}{c}\\ (d)\;\;& \frac{ab}{b+c}&\\ \end{flalign} Explanation :  \begin{flalign} Since \; DE\;&\Vert \; AB \; we \;can \;similar \; \triangle \; find \;the\; length&\\ \end{flalign} Using the properties of basic proportionality theorem we have  \begin{flalign} &\triangle \; CDE \; \sim \; \triangle \; CAB \ \end{flalign} \[\Rightarrow \; \frac{CE}{CB} =\frac{DE}{AB}\] \[OR \; \frac{CE}{BE + EC} =\frac{DE}{AB}\] \[\Rightarrow \; \frac{c}{b+c} =\frac{x}{a}\] \[OR \; \; {x} =\frac{ac}{b+c}\;...by \; cross \; multiplication\]   Guess the Option and comment below   👇

MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041)  Question 7   A point (x,y) is at a distance of 5 units from the origin. How many such points lie in the third quadrant? (a) 0  (b) 1  (c) 2  (d) infinitely many Explanation :  Distance between points P(x1,y1) and 𝑄(x2,y2) . As we know that formula of d istance between points P and 𝑄 will be  \[\sqrt{(x2 – x1)^2 + (y2 – y1)^2} \] Given that the point is at a distance of 5 units from the origin (0,0) we have \[\Rightarrow  \sqrt{(x-0)^2 + (y-0)^2} = \; 5,-----(a) \] \[OR \; \Rightarrow  \sqrt{(x)^2 + (y)^2} = \; 5 \] Squaring both sides to eliminate the square root \[ OR \; \Rightarrow \ x^2 \; + \;y^2 \; = \; 5^2\] ah! t his equation represents a circle with radius 5. As we know in the third quadrant, both x and y are negative(-,-)   If we substitute 𝑥 = −𝑎 and 𝑦 = −𝑏 into the equation, where a and b are positive values \[\therefore \ a^2 \; + \;b...

MATH SAMPLE QUESTION PAPER Class X Session 2023-24 MATHEMATICS STANDARD (Code No.041) Question 6 What is the ratio in which the line segment joining (2,-3) and (5, 6) is divided by x-axis? (a) 1:2  (b) 2:1  (c) 2:5  (d) 5:2 Explanation :  Lets understand first t he equation of the line passing through two points (x1,y1) and (x2,y2). \[\Rightarrow  (y-y1) = \frac{y2-y1}{x2-x1}\times (x-x1), -------(a) \] lets consider  two points  𝑃 and  𝑄 are  arbitrary  \[ \therefore 𝑃(2,−3) \; and \; 𝑄(5,6) \] similar to \[ 𝑃(x1, y1) \; and \; 𝑄(x2,y2) \] Now equation (a) becomes  \[\Rightarrow  (y-(-3) = \frac{6-(-3)}{5-2}\times (x-2), -------(b) \] \[ \therefore (y+3) = \frac{6+3}{5-2}\times (x-2) \] \[\therefore (y+3) = \frac{9}{3}\times (x-2) \] \[\therefore (y+3) = {3}\times (x-2) \] \[\therefore (y+3) = {3x-6} \] \[\; or \; y= {3x-6 -3} \] \[\; or \; y= {3x-9} ,----(c) \] To find the y-coordinate of the point where the line i...